=Graphene=
From my understanding, the emergent Dirac particle is seen in Graphene. In particular, one can read from any introduction of Graphene (google it), we know that:
1) There are emergent Dirac particles (up to some flavor number) in Graphene (for the gapless without external magnetic field)
2) it exhibits the (n+1/2) quantum Hall effect of Dirac fermion (for the gapped phase if we turn on external magnetic field),
There is a multiple of 4 or some even integer for the flavors of Dirac particle due to spin and valley degeneracy.
e.g.
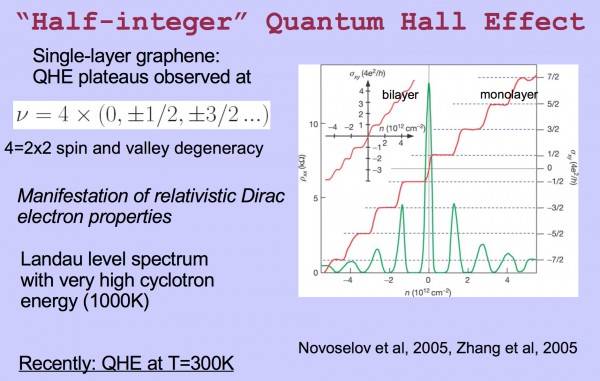
=1/2-filled Landau level=
Son proposed an interesting composite fermion picture as Dirac particle (vortex) distinct from the usual flux attachment procedure: http://journals.aps.org/prx/pdf/10.1103/PhysRevX.5.031027. and a related question is asked: http://physicsoverflow.org/37621/composite-fermions-attachment-relativistic-emergent-fermion.
Similarly,
1) There is an emergent composite Dirac particle (a single flavor). (For the gapless metallic phase, where the background magnetic field at 1/2 filled Landau level cancels out with effective flux of emergent gauge field.)
2) It emerges from the (0+1/2) un-quantized metallic Hall effect for non-relativistic electrons / fermions (for the gapless phase with a specific ratio of external magnetic field B and electron/fermion density ρ).
Question: Naively the emergent Dirac particles occur in two systems. There seemed to be some similarity in certain way, except that the Graphene is an anomaly-free system (multiple integer flavor of Dirac nodes), but the 1/2-filled Landau level is anomalous in some way due to the projection to the lowest Landau-level (a single integer Dirac nodes). (Anomaly in the sense of ABJ, t Hooft, parity anomalies, etc in HEP physics.) So:
1) Are there more similarity or difference between the two systems?
2) can one motivate the physics of Son's composite Dirac fermion from Graphene?
3) Why it takes so long to propose a theory of **composite Dirac fermion** from Son, even though people know that the **composite fermion in terms of flux attachment** (at least since 1989) for decades long? Doesn't Graphene also provide some hint for this possibility of **composite Dirac fermion**?
 Q&A (4905)
Q&A (4905) Reviews (206)
Reviews (206) Meta (439)
Meta (439) Q&A (4905)
Q&A (4905) Reviews (206)
Reviews (206) Meta (439)
Meta (439)