If one of the two analyzed energy types is zero, then there will be no significant difference between a Langrangian and the underlying Hamiltonian that solves the stream-lines of the recent found N-soliton solutions of the Navier Stokes d.e. Please do see also the following publication cited as
[1] R. Meulens , "A note on N-soliton solutions for the viscid incompressible Navier–Stokes differential equation", AIP Advances 12, 015308 (2022) https://doi.org/10.1063/5.0074083
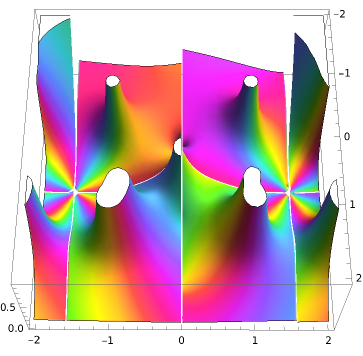
e.g. the benchmark driven-lid cavity fluid dynamical problem is a problem without significant effect of the potential energy. So in this case would the Langrangian be equivalent with the Hamiltonian. Below the 3d-lump (geometric sum of all solutions or a rational) solution of this problem found in [1]
 Q&A (4895)
Q&A (4895) Reviews (204)
Reviews (204) Meta (439)
Meta (439) Q&A (4895)
Q&A (4895) Reviews (204)
Reviews (204) Meta (439)
Meta (439)