At the end of my second answer to the post on Q&A, I mentioned possibilities of improving convergence of the asymptotic series with "re-expanding the searched function in powers of less growing functions " f(x) than powers of x (or g). These possibilities reduce the "impact" of the "uniqueness theorem" to the problem of approximating functions in practice.
Here I am again approximating the toy integral E(x), but this time I decided to use a simple function f(x)=x/(1+k⋅x), which has a coefficient k to adjust in order to nullify the term ∝f(x)3 in the expression E(x)≈1−f(x)+a(k)⋅f(x)2−b(k)⋅f(x)3.
Unfortunately (or fortunately?), the coefficient b(k) cannot be nullified for certain series. However it can be minimized. For E(x) such a k is equal to 2, bmin=2 and a(k=2)=0 The corresponding curves are given in Fig. 4 (I extended the axis x to x=5):
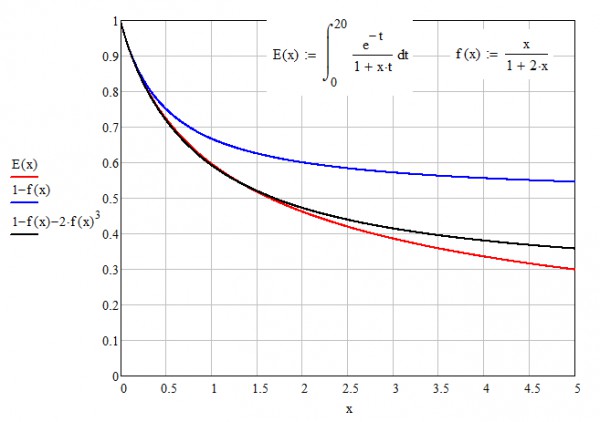
Fig. 4.
Precision of extrapolation of E(x) into the region of finite x is drawn in Fig. 5:
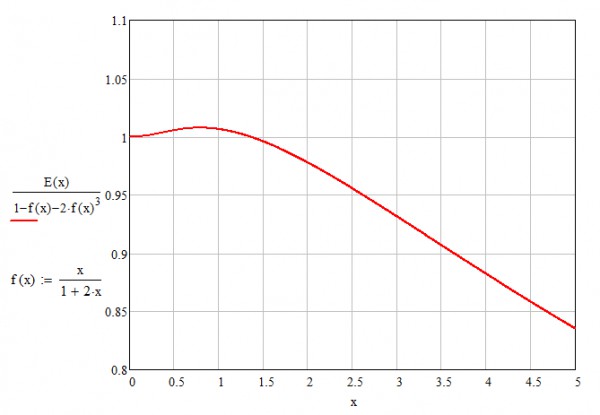
Fig. 5.
We can conclude that in order to successfully extrapolate the searched function E to big x, we have to choose such a function f(x), which provides sufficiently small coefficients or, better, smaller and smaller terms in the new expansion of E(x) (banality).
This conclusion is also supported with the next two figures (Fig. 6, 7), where the searched function is I(x)/√π and the extrapolating series are built in powers of a similar function f(x)=0.75x/(1+k⋅x). Here bmin=30.14, k=4.375, and a=0:
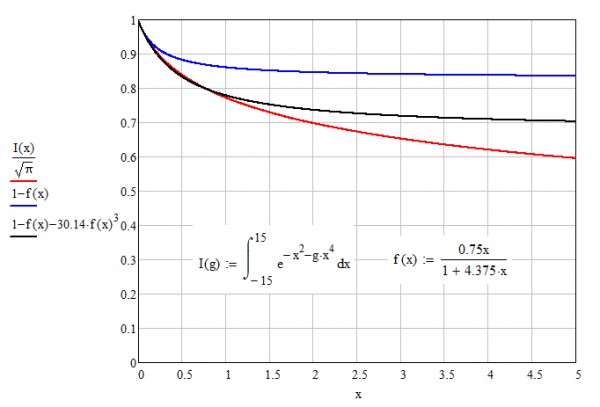
Fig. 6.
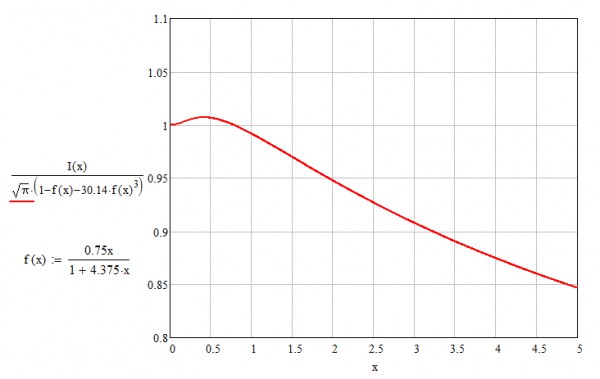
Fig. 7.
When the number of known terms is small, I think this constructive way of extrapolating functions may be rather practical. One bypasses the "uniqueness theorem" and arrives at a decent approximation.
Finally, the ground state energy E0(λ) of the anharmonic oscillator in QM (anharmonicity λx4) has also a divergent series: E0(λ)≈0.5(1+1.5λ−5.25λ2+41.625λ3), which can be transformed into a series in powers of f(λ)=1.5λ/(1+3.5λ). It gives a good extrapolation of E0(λ) (error ≤±1.5% within 0≤λ≤1, Fig. 8), unlike the original series (the exact curve was taken from here http://arxiv.org/pdf/quant-ph/0305128.pdf):
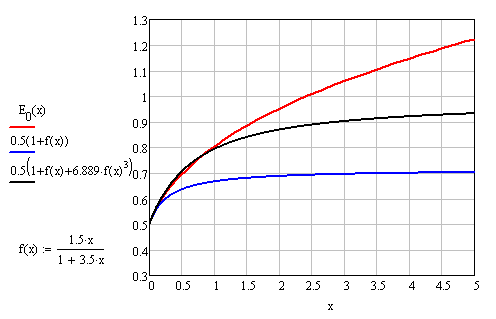
Fig. 8. The ground state energy E0(λ) of 1D anharmonic oscillator.
Thus, my idea was not too stupid as it allowed to extrapolate the asymptotic (divergent) series in the region of big  with a reasonable accuracy.
with a reasonable accuracy.
The moral is the following: if you want to have a “convergent” series, then build it yourself and enjoy.
 Q&A (4912)
Q&A (4912) Reviews (205)
Reviews (205) Meta (440)
Meta (440) Q&A (4912)
Q&A (4912) Reviews (205)
Reviews (205) Meta (440)
Meta (440)