Why does a spontaneously broken charge operator create exactly a one-Goldstone state?

Question

It is commonly claimed [2] that, if $j^0$ is a charge (density) that generates a spontaneously broken symmetry transformation, then

$$j^0|\text{VAC}\rangle=|\text{1-Goldstone}\rangle\cdots(1).$$

It can be shown (cf. Weinberg Vol2, chap 19, equation (19.2.34)), that $j^0|\text{VAC}\rangle$ has nonzero overlaps with 1-Goldstone states [1], but how can I see it's not, e.g., a supeposition 1-Goldstone and 2-Goldstone states? Or is equation (1) meant to be taken as a defining formula for 1-Goldstone state? In any case, there seems to be another paradox: it can also be shown (cf. Weinberg Vol2, chap 19, equation (19.2.35)), that  $\phi|VAC\rangle$ has nonzero overlaps with 1-Goldstone states, where $\phi$ is the scalar field that acquires VEV in the model[1]. However, by Noether's theorem, $j^0$ is a quadratic function of $\phi$ and $\pi$ ($\pi$ being the canonical conjugate of $\phi$),  and if $\phi$ has a mode expansion at all, wouldn't $j^0$ have to contain a term that creates two particles?

Furthermore, in demonstrating the non-existence of parity doubling of hadronic spectrum, it is claimed

$$j^0|h\rangle=|h, \text{1-Goldstone}\rangle\cdots(2),$$

where $|h\rangle$ is a 1-hadron state. Even if I take for granted that $j^0$ creats a 1-Goldstone when acting on vacuum, it's still not clear to me why it does not do anything to the hadron at all.

---

[1] But note in Weinberg's proof, nowhere did he explicitly defined what a 1-Goldstone state is. He only loosely defined 1-particle state as a state of which momentum is the only continuous index, and 1-Goldstone as a massless 1-particle state with nonzero overlap with both $j^\mu|\text{VAC}\rangle$ and $\phi|\text{VAC}\rangle$.

[2] For example, M. Schwartz, Quantum field theory and standard model

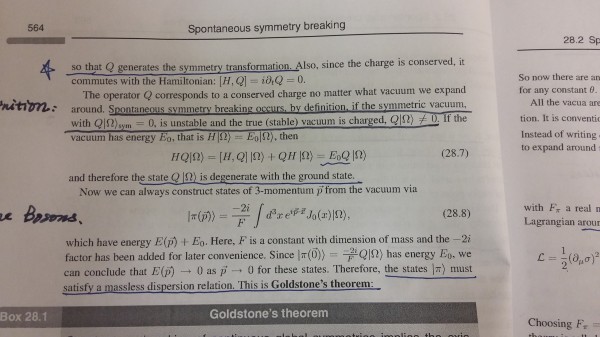
Also, Weinberg Vol 2, when talking about nonexistence of hadronic parity doubling:

Comments

This is difficult to answer as the fields make sense only as interactive fields, about very little is known when one asks too precise questions. Where is (1) claimed?

By the way, it should be "cf." and "e.g.,", not "c.f." and "e.g,"

---

To your note [1]: Weinberg defines after (19.2.8) what a Goldstone boson is - a pole in the effective action corresponding to a massless mode of the second derivative of the potential. Corresponding to each such pole is an asymptotic particle (Peskin-Schroeder, end of Section 11.5) that participates in the S-matrix and defines a Hilbert space of asymptotic 1-boson states. Being massless, these states cannot decay, hence this 1-particle space is preserved by translations, hence agrees with the space of nonasymptotic 1-boson states.

---

@ArnoldNeumaier, I just added two references for equation (1) (and corrected c.f and e.g, of course :D)

about footnot [1], in his second proof of Goldstone theorem (the non-effective-potential, current-algebra-like approach), he writes down single Goldstone states such as $|B\rangle$, but without writing its full relation with field operators, except some partial information such as (19.2.34) and (19.2.35).